Quy tắc cộng: \(\overrightarrow {BA} + \overrightarrow {AD} = \overrightarrow {BD} \) với B, A, D bất kì. Bước 1. Phân tích và giải Giải bài 6 trang 92 SGK Toán 10 tập 1 – Cánh diều – Bài 5. Tích của vecto với một số. Cho ABCD là hình bình hành. Gọi G là trọng tâm của tam giác ABC….
Đề bài/câu hỏi:
Cho ABCD là hình bình hành. Đặt \(\overrightarrow {AB} = \overrightarrow a ,\overrightarrow {AD} = \overrightarrow b .\) Gọi G là trọng tâm của tam giác ABC. Biểu thị các vecto \(\overrightarrow {AG} ,\overrightarrow {CG} \) theo hai vecto \(\overrightarrow a ,\overrightarrow b .\)
Hướng dẫn:
Quy tắc cộng: \(\overrightarrow {BA} + \overrightarrow {AD} = \overrightarrow {BD} \) với B, A, D bất kì.
Bước 1: Biểu diễn vecto \(\overrightarrow {BD} \) theo hai vecto \(\overrightarrow a ,\overrightarrow b .\)
Bước 2: Biểu diễn vecto \(\overrightarrow {BG} \) theo hai vecto \(\overrightarrow a ,\overrightarrow b \) dựa vào đẳng thức \(\overrightarrow {BG} = \frac{1}{3}\overrightarrow {BD} \)
Bước 3: Biểu thị các vecto \(\overrightarrow {AG} ,\overrightarrow {CG} \) theo vecto \(\overrightarrow {BG} \) và \(\overrightarrow a ,\overrightarrow b .\)
Lời giải:
Cách 1:
Gọi O là giao điểm của AC và BD.
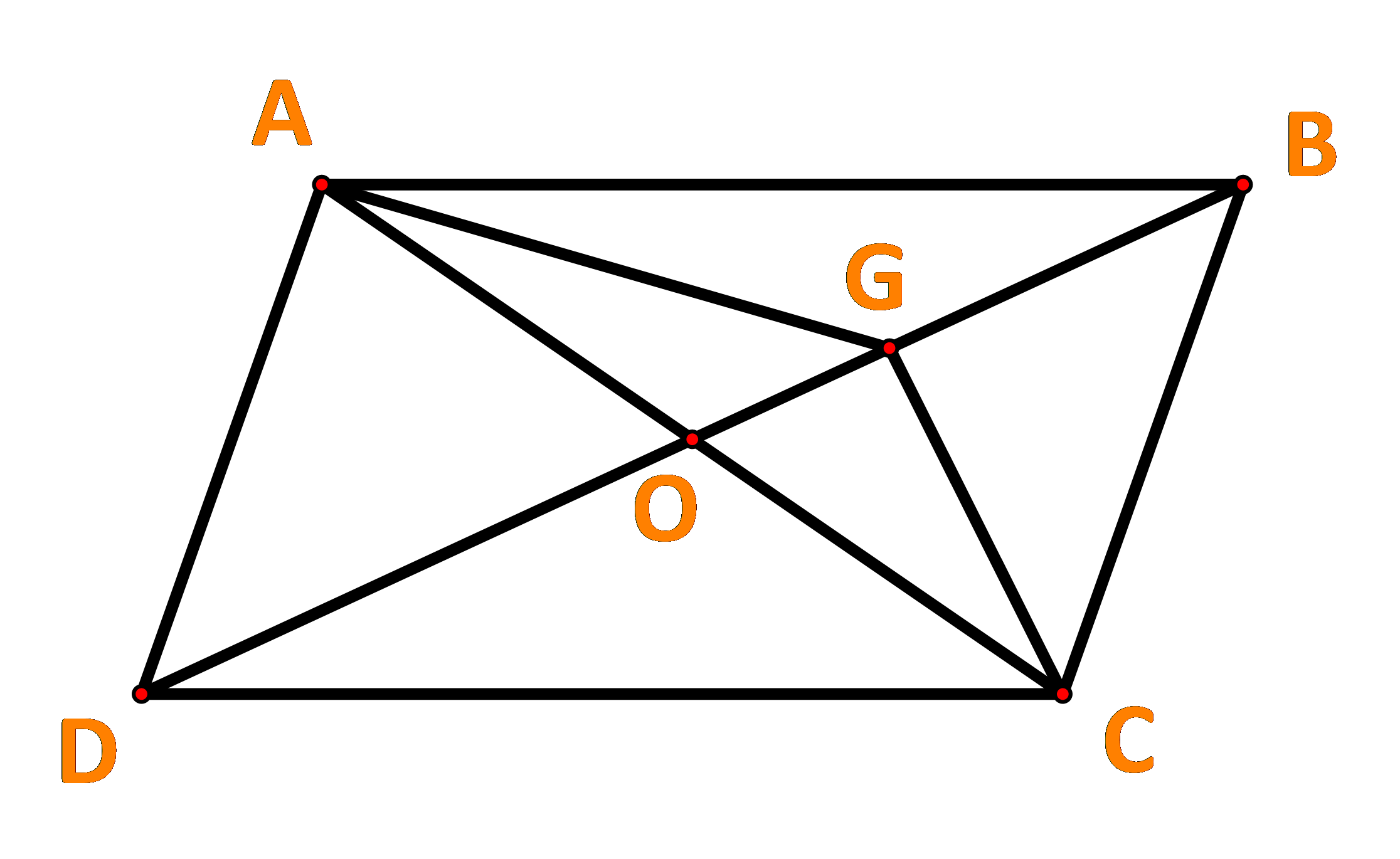
Ta có:
\(\begin{array}{l}\overrightarrow {AG} = \overrightarrow {AB} + \overrightarrow {BG} = \overrightarrow a + \overrightarrow {BG} ;\\\overrightarrow {CG} = \overrightarrow {CB} + \overrightarrow {BG} = \overrightarrow {DA} + \overrightarrow {BG} = – \overrightarrow b + \overrightarrow {BG} ;\end{array}\)(*)
Lại có: \(\overrightarrow {BD} =\overrightarrow {BA} + \overrightarrow {AD} = – \overrightarrow a + \overrightarrow b \).
\(\overrightarrow {BG} ,\overrightarrow {BD} \) cùng phương và \(\left| {\overrightarrow {BG} } \right| = \frac{2}{3}BO = \frac{1}{3}\left| {\overrightarrow {BD} } \right|\)
\( \Rightarrow \overrightarrow {BG} = \frac{1}{3}\overrightarrow {BD} = \frac{1}{3}\left( { – \overrightarrow a + \overrightarrow b } \right)\)
Do đó (*) \( \Leftrightarrow \left\{ \begin{array}{l}\overrightarrow {AG} = \overrightarrow a + \overrightarrow {BG} = \overrightarrow a + \frac{1}{3}\left( { – \overrightarrow a + \overrightarrow b } \right) = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\\\overrightarrow {CG} = -\overrightarrow b + \overrightarrow {BG} = -\overrightarrow b + \frac{1}{3}\left( { – \overrightarrow a + \overrightarrow b } \right) = – \frac{1}{3}\overrightarrow a – \frac{2}{3}\overrightarrow b ;\end{array} \right.\)
Vậy \(\overrightarrow {AG} = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\;\overrightarrow {CG} = – \frac{1}{3}\overrightarrow a – \frac{2}{3}\overrightarrow b .\)
Cách 2:
Gọi AE, CF là các trung tuyến trong tam giác ABC.
Ta có:
\(\overrightarrow {AG} = \frac{2}{3}\overrightarrow {AE} = \frac{2}{3}.\frac{1}{2}\left( {\overrightarrow {AB} + \overrightarrow {AC} } \right) = \frac{2}{3}.\frac{1}{2}\left[ {\overrightarrow {AB} + \left( {\overrightarrow {AB} + \overrightarrow {AD} } \right)} \right] \\= \frac{1}{3}\left( {2\overrightarrow a + \overrightarrow b } \right) = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b \)
\(\overrightarrow {CG} = \frac{2}{3}\overrightarrow {CF} = \frac{2}{3}.\frac{1}{2}\left( {\overrightarrow {CA} + \overrightarrow {CB} } \right) = \frac{2}{3}.\frac{1}{2}\left[ {\left( {\overrightarrow {CB} + \overrightarrow {CD} } \right) + \overrightarrow {CB} } \right] = \frac{1}{3}\left( {2\overrightarrow {CB} + \overrightarrow {CD} } \right) = \frac{1}{3}\left( { – 2\overrightarrow {AD} – \overrightarrow {AB} } \right) = – \frac{1}{3}\overrightarrow a – \frac{2}{3}\overrightarrow b \)
Vậy \(\overrightarrow {AG} = \frac{2}{3}\overrightarrow a + \frac{1}{3}\overrightarrow b ;\;\overrightarrow {CG} = – \frac{1}{3}\overrightarrow a – \frac{2}{3}\overrightarrow b .\)